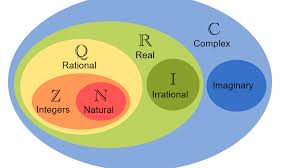
Complex numbers are excellent instruments for mathematical modeling, allowing for the description of different phenomena in a cohesive framework. They are used to solve differential equations, investigate fractals, and provide elegant solutions to problems that appear insurmountable using only real numbers.
- Teacher: collins kisang

What is coordinate geometry? In coordinate geometry, graphs and coordinates are used to find information about different geometric figures. A definition of coordinate geometry must include a description of the coordinate plane. The coordinate plane, also called the coordinate graph, is a grid system created by the intersection of two number lines, called axes. The horizontal number line is the x-axis, and the vertical number line is the y-axis. These axes intersect at a point called the origin. See the labeled diagram of a coordinate plane below.
 |
- Teacher: collins kisang
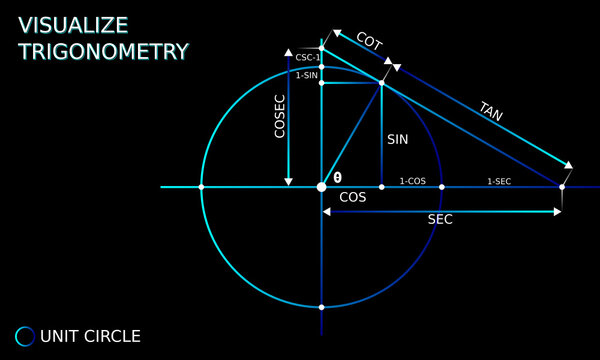
I In Mathematics, the hyperbolic functions are similar to the trigonometric functions or circular functions. Generally, the hyperbolic functions are defined through the algebraic expressions that include the exponential function (ex) and its inverse exponential functions (e-x), where e is the Euler’s constant. Here, we are going to discuss the basic hyperbolic functions, its properties, identities, and examples in detail.
- Teacher: collins kisang

The student studies principles of differential calculus (i.e. Cartesian geometry, graphing, functions, slope, limits, continuity, derivative), and applies rules (i.e. power, sum, product, quotient, chain) to calculate the derivative of various types of functions.
- Teacher: collins kisang